1. Compare the calculated and measured equivalent resistance values between the nodes A and B for three circuit configurations given below. Choose your own resistors. (Table).
- The following two tables contain data about the resistors for the three circuit configuration diagrams previously shown.
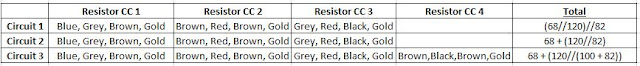 |
| Table 1: Resistor Color Codes, and calculation work. |
 |
| Table 2: Resistance calculation total from table above, compared to DMM measured resistance. |
2. Apply 5V on a 120 Ω resistor. Measure the current by putting the multimeter in series and parallel. Why are they different?
- In series the DMM says that the resistor has 40mA of current flowing through it, however in parallel the circuit has 0.08mA flowing in it. The difference between the two is the amount of current that runs through the multimeter. When the multimeter is in series, all of the current must pass through the multimeter. When the multimeter is in parallel, the current is divided between the multimeter leads and the resistor. The multimeter will only measure the current that passes through it, and not the circuits total current.
3. Apply 5 V to two resistors (47 Ω and 120 Ω) that are in series. Compare the measured and calculated values of voltage and current values on each resistor
 |
| Table 3: DMM measurement difference of voltages and currents between resistors set in series. |
Since the resistors are in the same loop of the circuit, they will have the same current. However, since the the resistors values are different, to satisfy Ohm's law (V=IR), the voltage must be different across the resistors since they have the same current.
4. Apply 5 V to two resistors (47 Ω and 120 Ω) that are in parallel. Compare the measured and calculated values of voltage and current values on each resistor.
 |
| Table 4: DMM measurement difference of voltages and currents between resistors set in parallel. |
5. Compare the calculated and measured values of the following current and voltage for the circuit below: (breadboard photo)
a.) Current on 2 kΩ resistor
 |
| Photo 1: Circuit layout shown on a breadboard |
a.) The measured current across the 2kΩ resistor was 1.95mA. The calculated current for the 2kΩ resistor was 1.984mA.
 |
| Figure 3: We converted the circuit into one resistor and used mesh analysis to find the current. The current of this loop will be equivalent to the current of the bottom loop in the full circuit. |
b.) The measured voltage for the bottom 1.2 kΩ resistor is 0.812V and the measured voltage for the right side 1.2 kΩ resistor is 0.690V. The calculated voltage for the bottom 1.2 kΩ resistor is 0.833V and the calculated voltage for the right side 1.2 kΩ resistor is 0.7032V.
6. What would be the equivalent resistance value of the circuit above (between the power supply
nodes)?
- The equivalent resistance between the two nodes of the circuit was 2.52kΩ. This was calculated by taking (2kΩ+(1.2kΩ//(100Ω+(1.2kΩ//1kΩ)))+100Ω)= 2519.7Ω.
7. Measure the equivalent resistance with and without the 5 V power supply. Are they different? Why?
- The resistance values are the same with or without the power running through the circuit. The values are the same because the multimeter sends its own voltage through the circuit to test the resistance. It doesn't matter whether or not the circuit is hot.
 |
| Figure 7: shows the resistance of the circuit with 5V running through it. |
- A potentiometer works by adjusting the resistance within itself to control the voltage output. The first pin is for voltage in and the opposite side pin is for ground. This is why the resistance across these two pins is always 10k. The middle pin is for output voltage which is controlled by the knob. The video attached shows this in action.
Video 1: Explains the terminal pin-out for a potentiometer
9. What would be the minimum and maximum voltage that can be obtained at V1 by changing the knob position of the 5 KΩ pot? Explain.
- This circuit contains only one resistor. This means that the voltage drop across the resistor must always be equivalent to the input voltage of, in this case, 5V. By adjusting the potentiometer's resistance, the only thing that will change is the current passing through the circuit.
Video 2: Shows how the position of the knob can affect the voltages at different locations
- The voltages V1 and V2 are related because V2 is always less than V1. V1 is equivalent to the input voltage of 5V at all times. V2 will always be equal to V1 minus the voltage across the 1k resistor. As the resistance
11. For the circuit below, YOU SHOULD NOT turn down the potentiometer all the way down to reach 0 Ω. Why?
- Turning the potentiometer all the way down to 0 Ω will short the circuit and allow too much current to flow through the circuit.
Video 3: Demonstrates how a 330 Ω resistor and a 10 kΩ pot correspond with each others current values based on the pot's knob position.
- When the potentiometer is set to its max resistance, the current through the 1k resistor will be at its max value because there will be much less resistance for the current to flow through the right loop. As the resistance of the potentiometer is moved closer to 0, the current in the left loop will increase because there will be a decreasing resistance for the current to flow through the left loop. As the left loop's current increases, the right loop's must decrease. This is how a current divider works.
13. Explain what a voltage divider is and how it works based on your experiments.
- A voltage divider works by using two resistors in series to reduce and/or control the larger input voltage. The voltage from the potentiometer can be controlled to values ranging from 0V to the input voltage. This is demonstrated in question 10.
14. Explain what a current divider is and how it works based on your experiments.
- A current divider works by using two resistors in parallel to reduce and/or control the higher input current. By using a potentiometer as a variable resistor in between loop one and loop two, we are able to control the current within the loops to which ever current is needed. This is demonstrated in the circuit from question 11.







